1. Introduction

In 2020, the world encountered the outbreak of COVID-19 pandemic, which resulted in major energy upset across the world [1], as well the social life. A slight decrease in biofuels jobs from 2.5 million to 2.4 million was observed [2]. In reaction to this new challenge, the improvement of sustainable energy assets and renewable energy power system sources has shown its promising potential and effective strategy to meet the new energy demand. Globally, an increase of 30% in the connected capacity of solar energy was noted between 2019 and 2020. Japan, India and Republic of Korea were the largest contributing countries in favouring the growth of solar power energy [2]. Presently, in renewable generation portfolio, wind energy occupies a major share, while the connecting capacity of solar photovoltaic (PV) system is also increasing yearly throughout the world [3]. This growing exploitation of renewable energy sources (RES) such as wind and solar energy brings many challenges to power system operators. Besides the load variation and the stochastic nature of the RES, the integration of RES brings about a subsequent reduction in the system inertia by replacing the rotating masses of the generators with power electronics devices.  Hence, stability of the power grid is being disturbed with penetration of RES. Power electronics technologies play a predominant role in the connection of distributed generation and renewable energy sources into the power grid and are becoming more prevalent and growing rapidly as these applications are further integrated into grid-based systems [4]. The fast evolution of power electronics devices are mainly due to development of semiconductor switches such as insulated gate bipolar transistor (IGBT) [5]. Hence the importance of studying the impact of integrating renewable energy on the stability of the power system.

Transient stability analysis (TSA) can be studied in a time-domain simulation. The nonlinear behaviour of a power system dynamics can be solved mathematically using numerical integration of the nonlinear differential equations.  Time-domain simulation provides flexibility, and it can consider all components of the power system. Equal Area Criterion (EAC) method provides a direct method for transient stability. This method was first introduced in the 1930s and 1940s to assess the transient stability of the classical model of a SMIB system [6]. It is used to determine the maximum load which can be acquired by the system without exceeding the threshold limit. The principle of this method consists of the basis that when the rotor angle (δ) oscillates around the equilibrium point with constant amplitude, thus maintaining transient stability. This present research work is an extended paper from the IEEE AFRICON 2021 conference proceedings [7]. A similar model is considered for further analysis on the transient stability study using the EAC method. The transient stability margin of the system and the critical clearing time (CCT) or the critical clearing angle were also considered in the analysis. Both parameters are essential elements in the stability analysis. The critical clearing angle was obtained through modelling of the swing curve on MATLAB/Simulink while the critical clearing time was obtained through mathematical derivation.

This research paper explains the concept of the EAC with connection of renewable energy using the positive phase sequencing and interpretation of results obtained. The main objective of this research paper is to investigate on the behaviours of a power grid in terms of stability in presence of RES. The method for developing the EAC consists of reducing the multi machine system into two sets, where one set is considered as the critical cluster while the other set is comprised of the remaining machines. CCT calculation for the stability studies in the presence of renewable energy contribute to knowledge of this research work.

This research paper is categorized into the background, theory, methodology, results and conclusions.

2. Background

      This section describes the background works in the transient stability field. In [7], the authors studied the stability in terms of rotor angle analysis with connection of RES.  The model consisted of synchronous generators and a solar PV system connected in an IEEE 9-bus arrangement. The investigation of the study was monitored under steady state condition and under transient condition such as a 3-phase fault. The result was compared to a conventional IEEE 9-bus arrangement. The author concluded that the integration of solar PV system brought an enhancement in the transient nature of the model under investigations due to a lower rotor angle deviation.

      In [8], the authors discussed a unified approach on transient stability assessment of a multi machine power system with the initial objectives set as establishing the fundamentals of extended equal area criterion (EEAC), intrinsic limitations, basic formulations and applications to actual working conditions operating within a general threshold security limit. The proposed method consisted of using the equal area criterion as applied to a two-machine system. The EEAC thus established, gives the visual and analytical interpretation for transient stability analysis and sensitivity assessment. However, the limitation of the research is that it does not include any control, which could be applied to the system to enhance the transient stability. Other methods of applications of Artificial Intelligence (AI) to the system may enhance the transient stability particularly in presence of renewable energy.

      The authors in [9] made use of a phase sequence exchange (PSE). The aim of the paper was to assess the efficiency of the PSE in a multi-machine system. The method consisted of installing two PSE devices positioned in a multi-machine system, with the first one installed at the outlet of each generator, and the second was the interconnected lines between the system. EEAC was used, and a control method was then proposed. It was observed that the use of the control methods maintained the synchronization, thus ensuring the system integrity and power quality. The efficiency of the PSE was checked against Kundur’s two-area four-machine power system and the IEEE 39-bus system and compared with other emergency control methods.

      In [10], the authors provided an overview of a revisited EAC with the aim of illustrating the different types of transient stability under occurrence conditions, namely type I, type II, type III and type IV. Four types of variables were discussed on the EAC, P_m, P_b, P_d, P_p where P_m is the mechanical input power while P_b, P_d, P_p are corresponding maximum power during the pre-fault, during fault and post fault. Conditions of always stable even during the fault and unstable states were investigated when P_d<P_p and P_d>P_p. Type I was typical and widely accepted as common sense in the transient stability analysis. The study proved relevant to the development of relay protection for enhancing and maintaining the power system stability.

The authors in [11] investigated on the transient rotor angle stability during extreme conditions such as three-phase fault, the disconnection of generators, change of weather due to its stochastic nature and partial shutdown of PV generation [12]. Transient stability rating inclusive of the swing motion and damping are determined due to change of angular speed, frequency, and power output. Other factors such as critical clearing time (CCT) and loadability have been also studied in [13]. It was concluded that the transient stability rating of the grid was dependent on the extent of PV connection and type of transient events induced in the system.

In [14], the authors studied the impact of integrating photovoltaic generation penetration and together with the use of Flexible AC transmission (FACTS) devices such as STATCOM on the transient stability of a single machine infinite bus (SMIB) system based on the rotor angle stability. The system model was created in MATLAB/Simulink software. It was concluded that with the penetration of solar PV along with the use of STATCOM was able to provide solutions to improve the behaviour of networks by damping the transient oscillations of the rotor angle for various fault conditions.

      The literature survey described above shows some research gaps in the field of transient stability study in presence of renewable energy using the EAC method. Application of artificial intelligence (AI) in the transient stability has also received little attention in research. It is expected that the use of AI will enhance the use of RES by appropriate prediction of fault. However, the use of AI will not be considered within this research paper and same will be considered as further works.  EAC will determine the critical clearing angle (CCA). The critical clearing time (CCT) will be determined using mathematical derivation and numerical integration. Both parameters are essential in the study of transient stability. The critical clearing angle is the maximum allowable change in the power angle δ before clearing the fault and without loss of synchronism. The critical clearing time is the maximum allowable time to remove a disturbance without interrupting the system integrity. To our knowledge, no studies of similar nature have been conducted for transient stability analysis.

3. Theory

This section explains the theory behind the single machine infinite bus (SMIB) and the equal area criterion (EAC).

3.1.  Single Machine Infinite Bus (SMIB)

The diagram in Figure 1 is the equivalent representation of a SMIB in a power system through a line of reactance X_e.

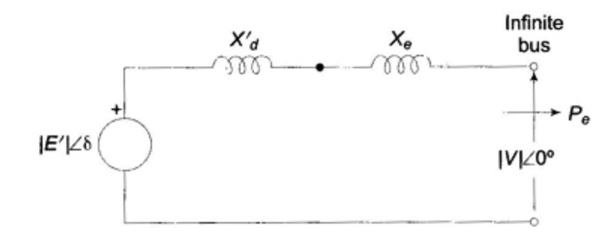
Figure 1: Arrangement of single machine

A generator operating at synchronous speed ω_m will develop an electromagnetic torque T_e and a corresponding electromagnetic power P_e.  The input torque developed by the prime mover at the generator shaft is T_i, then under steady state conditions, T_i = T_{e .}

However, under disturbances, the input power, P_i is no longer equal to the output power, P_e. This gives rise to an accelerating power, ΔP which is equivalent to the accelerating torque.

According to [15],

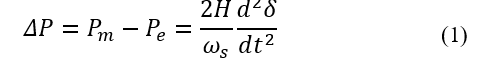
where

ΔP: Accelerating power (pu)

δ: Rotor angle (rad)

: Angular speed (rad/s).

H: Inertia (MJ/MVA)

P_m: Mechanical Power (pu)

P_e: Electrical Power (pu)

Figure 2: Two-Machine system

A two machines system operating at normal dynamic condition, i.e, steady state, the electrical power developed by the generator applications is equal to the power absorbed by the motor (network being lossless). Thus, at all time

 

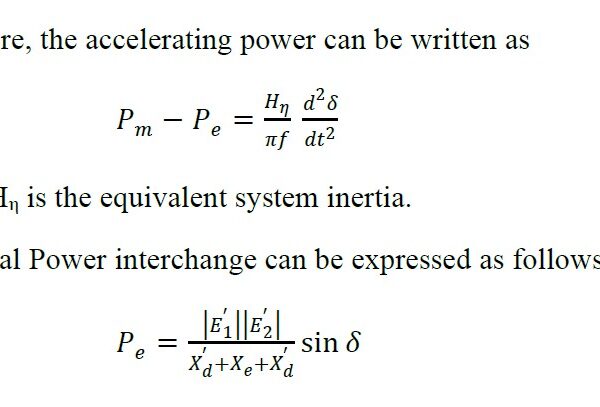
A two machines system has the same form of swing and power angle equations, as that of a single machine connected to an infinite bus [16, 17].

3.2.  Equal Area Criterion (EAC)

Stability assessment for a SMIB may be determined based on the rotor angle deflection. It is also possible to define the stability graphically using the power-angle diagram, which is known as the equal area criterion (EAC).

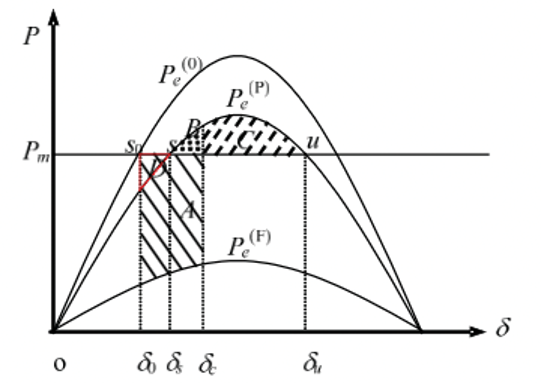
Figure 3: Power angle curve

According to [18], when

Area A < Area C: Stability is achieved within first swing operation.

Area A > Area C: System is not stable.

Area A = Area C: Machine is critically stable in the first swing.

Figure 4: IEEE 9-bus arrangement

In case of a multi machine system, when

When Area A < Area C: the system is operating synchronously in the first swing.

When Area A > Area C: the system is operating asynchronously in the first swing.

When Area A = Area C: the system is operating in a critically synchronous condition within the first swing.

3.3.  Methodology

The proposed system under study is comprised of an adapted IEEE 9-bus with the system frequency changed to 50 Hz. Figure 4 shows the arrangement of synchronous generators under investigation. The default values of the system’s impedances were used during the study.

The power angle curve can be developed based on the impedance of the generator and the transmission line, which is shown in Figure 5.

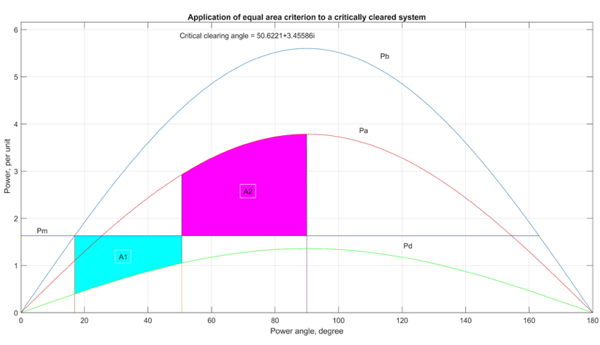
Figure 5: Power angle curve for an IEEE 9-bus arrangement

During the stability assessment of the system after the clearance of the fault, the accelerating energy (A1) and decelerating energy (A2) can be calculated using the principle laid down by [19],

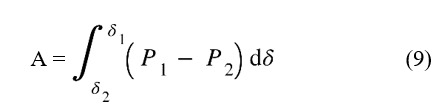
In the present condition,

A₂ > A₁ which implies stable state of the system.

      The critical clearing time of the system is calculated with integration of (7) above, which will result in (10) according to [20]

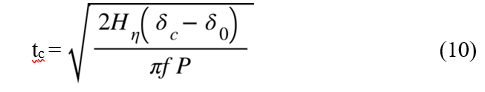
where t_c is the critical clearing time

H_η: Εquivalent inertia (MJ/MVA)

δ_c: critical clearing angle (°)

δ_o: angle at steady state (°)

f: frequency (Hz)

P: Power (MVA)

      The equivalent inertia H_η can be determined using the R method which is described in [21]. The resulting critical time for the generation mix of synchronous generator is 0.4 s for an estimated inertia of 33.125 MJ/MVA.

      During the integration of the solar PV system, generator G2 is displaced from the grid as per the following arrangement in Figure 6.

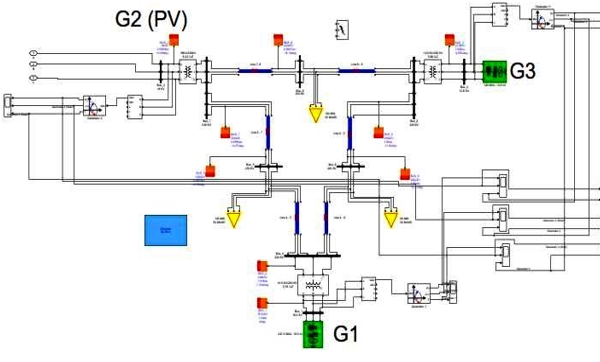
Figure 6: Solar PV system connected in an IEEE 9-bus arrangement

      The first step in developing the power angle curve for this new arrangement, a single axis machine was considered, together with system impedance. The network was reduced to an equivalent circuit diagram by applying Thevenin’s theorem, which is given in Figure 7.

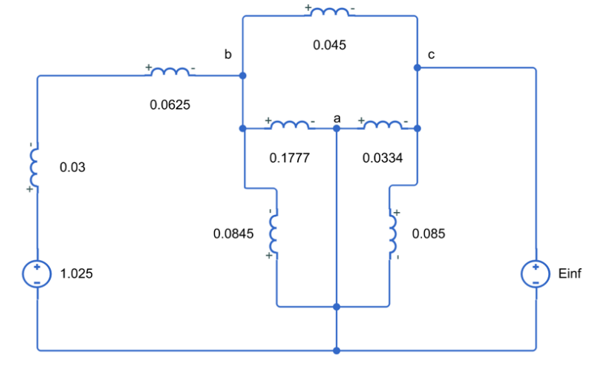
Figure 7: Equivalent circuit with solar PV integration

      The result of the power angle curve is shown in Figure 8.

Figure 8: Equal Area Criterion with connection of renewable energy

      The integration of solar PV system into the network has lowered the critical clearing angle from 50° to 43°. Using the principle as described above, it can be determined that A2 > A1, which confirmed the stable state of the system. Moreover, equation (9) in [22] was applied to determine the equivalent inertia. The estimated inertia was calculated using (11)

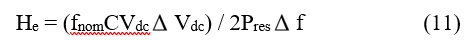
      According to [22], (V_dc) /f is a constant. Allowing a maximum change of frequency of 0.5Hz and a dc link voltage of 500 V, the constant can be taken at 1000. An estimated value of H_e = 6 MJ/MVA can be availed from the expression above. Upon substituting in (10) above, it is observed that the critical clearing time increased to 1.18 s.

4. Conclusion

As mentioned in the introduction, the exploitation of the solar energy is gradually increasing. It was demonstrated through this study that the system dynamics are impacted with the penetration of large-scale renewable generation. Thus, it is imperative to set the conditions for  the steady state and dynamic performance of a power system for different installation scenarios of renewable power plants such as photovoltaic (PV) ones. This study can prove useful to power system operators and policy makers when it comes to the decarbonization of the power grid and its stability concept.

This research paper described the equal area criterion method as a means of transient stability analysis. Similar method can be applied in presence of renewable energy such as solar PV system. The study concluded that with solar power generating system forming part of the generation mix, an improvement of the transient stability of the grid were noted due to a decrease in the critical clearing angle from 50° to 43°.

The paper also brought about an innovative method of estimating the critical clearing time based on an estimated inertia with presence of RES.  The critical clearing time was increased from 0.4s to 1.18s in the second test condition owing to the presence of solar PV system, thus confirming an enhancement in the transient stability. Further investigation needs to be worked on the application of AI to such model for fault prediction.

Conflict of Interest

The authors declare no conflict of interest.

Acknowledgment

The assistance given by Advanced Mechanical and Electrical Services Ltd. in the development of this research paper was greatly appreciated.

1. A.T. Hoang, S. Nižetić, A.I. Olcer, H.C. Ong, W.-H. Chen, C.T. Chong, S. Thomas, S.A. Bandh, X.P. Nguyen, “Impacts of COVID-19 pandemic on the global energy system and the shift progress to renewable energy: Opportunities, challenges, and policy implications,” Energy Policy, 154, 112322, 2021, https://doi.org/10.1016/j.enpol.2021.112322.
2. IRENA and ILO, Renewable Energy and Jobs – Annual Review 2021, International Renewable Energy Agency, International Labour Organization, Abu Dhabi, Geneva, 2021.
3. I.S. Jha, S. Sen, K. Bhambhani, R. Kumar, “Grid Integration of Renewable Energy Sources,” International Journal of Scientific and Technical Advancements (IJSTA), 1, 1-5, 2015.
4. M.E.T. Souza Junior, L.C.G. Freitas, “Power Electronics for Modern Sustainable Power Systems: Distributed Generation, Microgrids and Smart Grids—A Review,” Sustainability, 14, 3597, 2022, doi: 10.3390/su14063597
5. J.M. Carrasco et al., “Power-Electronic Systems for the Grid Integration of Renewable Energy Sources: A Survey,” IEEE Transactions on Industrial Electronics, 53, 4, 1002-1016, 2006, doi: 10.1109/TIE.2006.878356.
6. A. Bahmanyar, D. Ernst, Y. Vanaubel, Q. Gemine, C. Pache, P. Panciatici, “Extended Equal Area Criterion Revisited: A Direct Method for Fast Transient Stability Analysis,” Energies, 14, 7259, 2021, doi:10.3390/en14217259
7. A.A. Jhumka, R.T. F. Ah King, C. Ramasawmy, “Rotor Angle and Inertia Analysis in Presence of Renewable Energy Source,” 2021 IEEE AFRICON Conference, 1-6, 2021, doi: 10.1109/AFRICON51333.2021.9571011
8. Y. Xue, M. Pavella, “Extended equal-area criterion: an analytical ultra-fast method for transient stability assessment and preventive control of power systems,” International Journal of Electrical Power & Energy Systems, 11(2), 131–149, 1989, doi:10.1016/0142-0615(89)90021-5.
9. Y. Li, S. Huang, H. Li, J. Zhang, “Application of phase sequence exchange in emergency control of a multi-machine system,” International Journal of Electrical Power & Energy Systems, 121, 106136, 2020, doi:10.1016/j.ijepes.2020.106136.
10. Y. Sun, J. Ma, J. Kurths, M. Zhan, “Equal Area Criterion in power systems revisited,” Proc. R. Soc. A. 474:20170733, 2018. doi:10.1098/rspa.2017.0733
11. S. You, G. Kou, Y. Liu, X. Zhang, Y. Cui, M.J. Till, W. Yao, Y. Liu, “Impact of high pv penetration on the inter-area oscillations in the us eastern interconnection,” IEEE Access 5, 4361–4369, 2017, doi: 10.1109/ACCESS.2017.2682260.
12. S. Eftekharnejad, V. Vittal, G. T. Heydt, B. Keel, J. Loehr, “Impact of increased penetration of photovoltaic generation on power systems,” IEEE Transactions on Power Systems, 28(2), 893-901, 2013, doi: 10.1109/TPWRS.2012.2216294.
13. B., Tamimi, C., Cañizares, K. Bhattacharya, “System stability impact of large-scale and distributed solar photovoltaic generation: The case of Ontario, Canada,” IEEE Trans. Sustain. Energy 4(3), 680–688, 2013.
14. S. Fettissi, D. Labed, I. Labed, “Influence of High PV Penetration and STATCOM on Rotor Angle Stability of SMIB Transmission System,” Journal of Electrical Engineering & Technology, 849-857, 2018.
15. M. Pavella, D. Ernst, D. Ruiz-Vega, Transient stability of power systems: a unified approach to assessment and control, Springer, 2000.
16. P. Kundur, N.J. Balu, M.G. Lauby, Power system stability and control, McGraw-Hill. 1994.
17. L. Fang and Y. Ji-lai, “Transient stability analysis with equal area criterion directly used to a non-equivalent generator pair,” International Conference on Power Engineering, Energy and Electrical Drives, 386-389, 2009, doi: 10.1109/POWERENG.2009.4915162.
18. S. Paudyal, R. Gokaraju and M. Sachdev, “Application of equal area criterion conditions in the time domain for out-of-step protection,” IEEE PES General Meeting, 1-1, 2010, doi: 10.1109/PES.2010.5589303.
19. H.-D. Chiang, F.F. Wu, P.P. Varaiya, “A BCU method for direct analysis of power system transient stability,” IEEE Transactions on Power Systems, 9(3), 1194–1208, 1994, doi:10.1109/59.336079.
20. F.I. Khan, T. Aziz, “Transient Stability evaluation of a Solar PV Integrated Industrial Microgrid,” IEEE Region 10 Symposium (TENSYMP), 535-540, 2019, doi: 10.1109/TENSYMP46218.2019.8971169.
21. D. Zografos, M. Ghandhari, “Estimation of power system inertia,” IEEE Power and Energy Society General Meeting (PESGM), Boston, MA, USA, 1-5, 2016, doi: 10.1109/PESGM.2016.7741073.
22. R. Ghosh, N.R, Tummuru, B.S. Rajpurohit, A. Monti, “Virtual Inertia from Renewable Energy Sources: Mathematical Representation and Control Strategy,” IEEE International Conference on Power Electronics, Smart Grid and Renewable Energy (PESGRE2020), 1-6, 2020, doi: 10.1109/PESGRE45664.2020.9070733.

(Click Here)